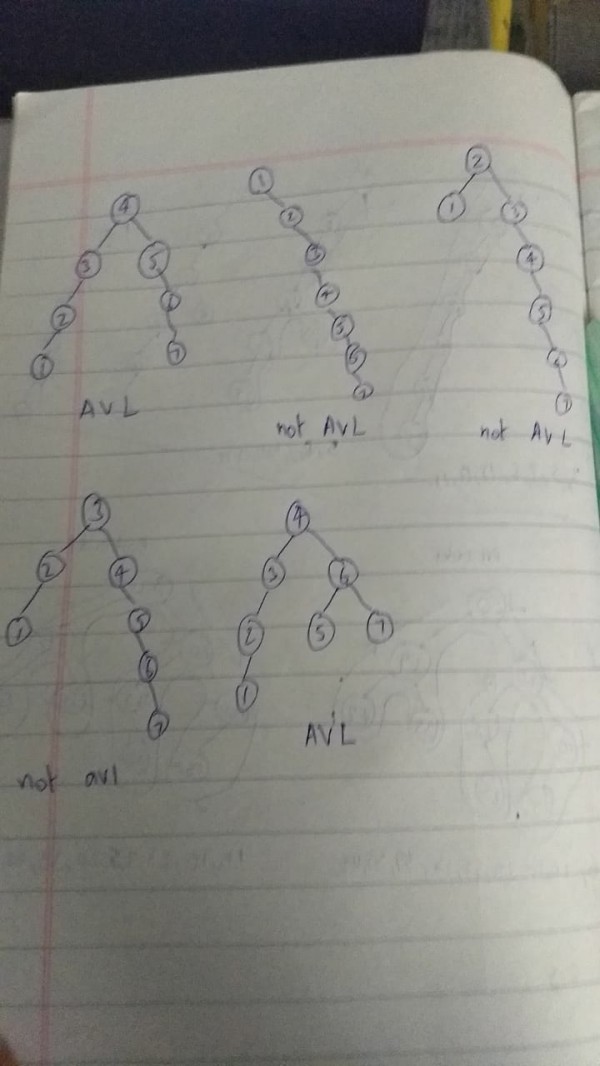
AVL tree is binary search tree with additional property that difference between height of left sub-tree and right sub-tree of any node can’t be more than 1
- If there are n nodes in AVL tree, minimum height of AVL tree is floor(log2n).
- If there are n nodes in AVL tree, maximum height can’t exceed 1.44*log2n.
1.44*log7 = 4 so maximum height can’t exceed 4
so to get max height if we keep Minimum number of nodes at each level there is chance to get maximum height
eg:at 1st level 2 elements one at right other at left(because we should satisfy avl property) similarly others at 2nd level 2 elements at 3rd level 2 elements
eg: