The given K-map is not standard as after "01" we have "10" and two variables are changing for consecutive column. This means it is not safe to merge adjacent $1s.$ By converting the K-map to standard form we get
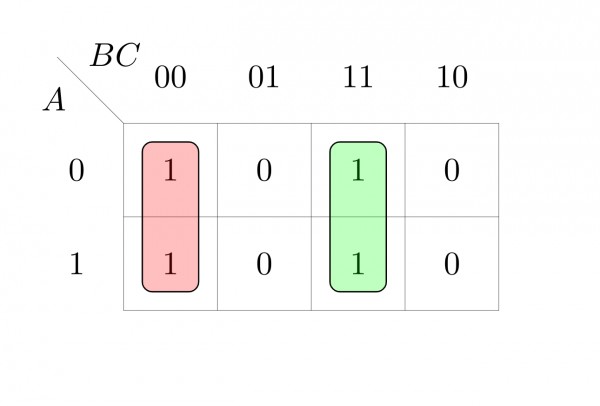
which gives
$BC + \bar B\bar C = B \text{ XNOR } C = B \odot C$
This can be represented as negation of $\text{XOR} = \overline{B \oplus C}$
Option C is correct.