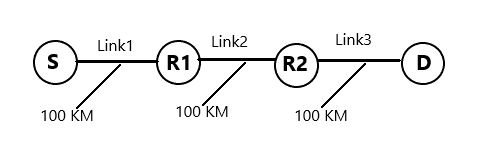
We have been given following info:
Signal Speed = 10^8 m/s
Bandwidth, BW = 1 Mbps = 10^6 bps
Propagation time, Tp = Link Distance/ Link Speed = (100x10^3)/(10^8) = 10^-3 sec = 1 ms.
[Propagation time is the time taken by packet to travel through the link]
Transmission time for 1 packet, Tt = Packet Size / BW = 1000 / 10^6 = 10^-3 sec = 1 ms.
[Transmission time is the time taken by source or router to process or put the data on Link]
Link filling time can be calculated as:
Pipeline Filling time
| Time |
L1 |
R1 |
L2 |
R2 |
L3 |
D |
| t=0 |
|
|
|
|
|
|
| t=1 |
P1 |
|
|
|
|
|
| t=2 |
P2 |
P1 |
|
|
|
|
| t=3 |
P3 |
P2 |
P1 |
|
|
|
| t=4 |
P4 |
P3 |
P2 |
P1 |
|
|
| t=5 |
P5 |
P4 |
P3 |
P2 |
P1 |
|
| t=6 |
P6 |
P5 |
P4 |
P3 |
P2 |
P1 |
This way time taken by packet P1 to reach Destination (D) = 6 ms
Now, as you can see, at every ms, 1 packet will reach D.
Therefore, time taken for remaining 999 packets = 999 ms.
So, total time taken to transmit 1000 packets = 999 + 6 = 1005 ms.
Hence Option (A) is correct answer.