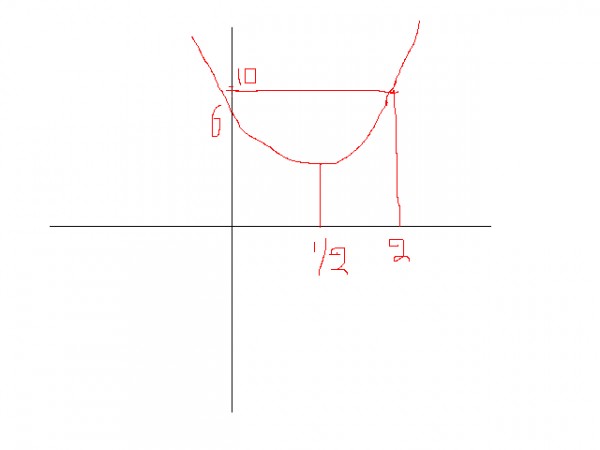
in such type of questions it's better to know the nature of curve
General case :- Let $f(x)=ax^{2}+bx+c$ where $a\neq0$
a) concave or convex
1) if $a>0$ then curve will open up (convex nature)
2) if $a<0$ then curve will open down (concave nature)
b) Nature of roots
Let $D=b^{2}-4ac$
1) if $D>0$ , then our curve will intersect $x-axis$ at two different points (means two different real roots)
2) if $D=0$ , then our curve will touch $x-axis$ at one point (means two same real roots)
3) if $D<0$ , then our curve won't touch or cut $x-axis$. Means either it will be completely above the $x-axis$(when $a>0$) or it will be entirely below the $x-axis$ (when $a<0$).Hence imaginary roots.
Original question
$f(x)=2x^{2}-2x+6$
1) $a=2$ , so $a>0$ means curve will be open up nature
2) $D=-44$ , so $D<0$ means our curve will be entirely above $x-axis$ , mean imaginary roots.
$\frac{\mathrm{d} y}{\mathrm{d} x}=4x-2=0$ , so at $x=\frac{1}{2}$ , we have Max/Min.
although from graph we can directly it would be minima at $x=\frac{1}{2}$ , yet another way is to know the nature of second derivative which is $\frac{\mathrm{d} (4x+2)}{\mathrm{d} x}=4$ , so $+ve$ means minima at $x=\frac{1}{2}$
So at that level from graph we can directly say , either graph will have maxima in $[0,2]$ at $x=0$ or $x=2$ , so just calculate the value at those points and see which one is max.