TIFR CSE 2014 | Part A | Question: 9

Question

Solve min $x^{2}+y^{2}$ subject to

$$\begin {align*} x + y &\geq 10,\\
2x + 3y &\geq 20,\\
x &\geq 4,\\
y &\geq 4.
\end{align*}$$

• A. $32$
• B. $50$
• C. $52$
• D. $100$
• E. None of the above

Answer 1

Answer: Option $(B)$ $50$

 $x\geq 4$ and $y\geq 4$ , So we can take both $x =5$ & $y = 5$

$x+y \geq 10,$ Satisfied , $5+5 = 10$

$2x + 3y \geq 20$. Satisfied.

This is in fact the minimum value.

Other options:

$4,4 \implies x+y \geq 10$ constraint fail

$4,5 \implies  x+y \geq 10$ fail

$6,4 \implies$ Sum $=52$ which is more than $50$ and hence cannot  be answer.

$7,3 \implies 49+9 = 58$ which is again greater than $50$ and hence cannot be the answer.

Comments

is brute force only possible way to solve such questions????

---

I think that condition 2 is redundant:

$\Rightarrow 2x+3y\geq 20$

$\Rightarrow 2x+2y+y\geq 20$

$\Rightarrow 2(x+y)+y\geq 20$ 

$\Rightarrow y\geq 0$ ..... (Using $x+y\geq 10$)

The question can be solved by using only first condition. Refer the link below to solve further 

https://socratic.org/questions/what-is-the-smallest-possible-value-of-the-sum-of-their-squares-if-the-sum-of-tw

Answer 2

$x^2 + y^2 = (x+y)^2 -2xy$

To minimize $x^2 + y^2$ we have to maximise $xy$.

Now, $x + y = 10$. So, we maximise $x(10-x)$.

We get $x = 5$ after maximising it.

So finally $x^2+y^2 = 5^2 + (10-5)^2=50$.

Answer 3

I think it's the easiest one,
approach - first we just break to the minimum conditions so every thing can be meet. minimum value such that every thing can satisfy is 5 , 5 which gives = 50,

now all the possiblities are decreasing one number and increasing one. but as u think . as u will decrease one number the value that will decrease will be less then the after effect of increasing the other number.
like 4 and 6 . value that decreases due to decreasing it from 5 is (25-16) = 9

but the increase in the value due to increasing 5 to 6 is (36-25)= 11 , so the best answer will be the mid point i.e 5,5 = 50

Answer 4

Here, given problem comes under the category of Constrained Non-linear Optimization.

Since, objective function is convex function, So, it is Convex Programming problem.

Here, objective function $x^2 + y ^2$ is called level curve or circular contour curve or constant cost contour.

Here, we get the feasible region as :

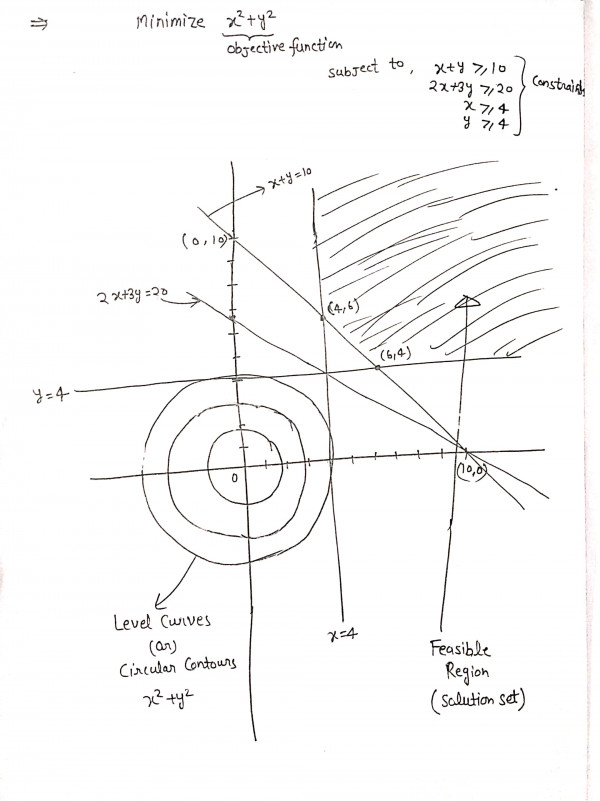
So, point $(x,y)$ for which $x^2 + y^2$ is minimum, lies in the feasible region(shaded region) which we have to find.

This circular contour $x^2 + y^2$ will grow and since, its nearest boundry of feasible region is a line segment whose end points are $(4,6)$ and $(6,4)$ and when this level curve $x^2 + y^2$ touches the line segment, we get the minimum value.

So, actually, we have to find the point of tangency of $x^2 + y^2$ and line $x+y = 10$

 

Here, Line passes points ‘O’ and ‘P’ is perpendular to line $x+y = 10$

So, if slope for line passes through O and P is $m_1 $ then $m_1 = \frac{k}{h}$

and slope of line $x+y =10$ is $m_2 = -1$

Since, both lines are perpendicular to each other.

So, $m_1 \times m_2 = -1$

$ \frac{k}{h} \times (-1) = -1$

$\Rightarrow h=k$

point $(h,k)$ satisfies the line $x+y =10$

So, $h+k =10$

$\Rightarrow 2h = 10$

$\Rightarrow h=5$ and so, $k=5$

Hence, for point $(5,5),$ $x^2 + y^2$ gets the minimum value which is $5^2 + 5^2 = 50.$

We can also use the idea of Lagrange Multiplier here.

Here, Lagrangian function is :

$L(x,y,\lambda) = x^2 + y^2 – \lambda(x+y-10)$

Now, $\frac{\partial L}{\partial x}$ at $x=x^*$ is $2x^* – \lambda$

$\frac{\partial L}{\partial y}$ at $y=y^*$ is $2y^* – \lambda$

and $\frac{\partial L}{\partial \lambda}= -x^* -y^* +10$

For optimality, $\frac{\partial L}{\partial x} = 0, $ $\frac{\partial L}{\partial y}=0$ and $\frac{\partial L}{\partial \lambda}= 0$

So, we have $x^* = y^* = \frac{\lambda}{2}$

and $x^* + y^* = 10$ which implies $\frac{\lambda}{2} + \frac{\lambda}{2} = 10$ $\Rightarrow \lambda = 10$

Hence, optimal point is $(x^*,y^*) = (5,5)$

Therefore, minimum value of $x^2 + y ^2$ is $50.$

 

For visualization :

https://www.desmos.com/calculator/vhplzfc3lb

https://www.desmos.com/calculator/jsx6dibkno