Register

UGC NET CSE | June 2019 | Part 2 | Question: 7

• edited by
6,045 views
4 votes
4 votes

How many cards must be selected from a standard deck of $52$ cards to guarantee that at least three hearts are present among them?

  1. $9$
  2. $13$
  3. $17$
  4. $42$
• edited by
User Avatar

1 Answer

5 votes
5 votes

In this the worst case will happen as follows :-

$1^{st}$ $13*3 = 39$ cards that we pick up turns out to be any one of daimond or spades or clubs but not hearts i.e.

 

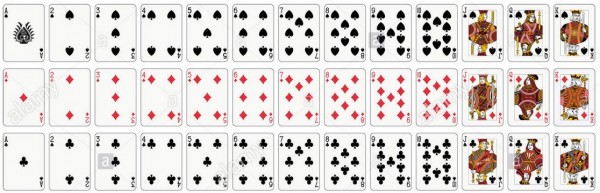
Now when we pick the next $3$ cards they are guaranteed to be hearts.($\because$ we have already picked cards from all other suits)

 

$\therefore$ We need to pick $13+13+13+3= 42$ cards in order to guarantee that there are atleast $3$ cards of hearts in the selected cards.

So option $4$ is the correct option.

• edited by
User Avatar
Voting & Accepting an answer helps improve the community
Answer:
← PreviousNext →
← Previous in categoryNext in category →

Related questions

2 votes
2 votes
2 answers
1
Arjun asked Jul 2, 2019
7,151 views
UGC NET CSE | June 2019 | Part 2 | Question: 2
How many ways are there to place $8$ indistinguishable balls into four distinguishable bins? $70$ $165$ $^8C_4$ $^8P_4$
How many ways are there to place $8$ indistinguishable balls into four distinguishable bins?$70$$165$$^8C_4$$^8P_4$
User Avatar
3 votes
3 votes
2 answers
2
Arjun asked Jul 2, 2019
5,813 views
UGC NET CSE | June 2019 | Part 2 | Question: 3
How many bit strings of length ten either start with a $1$ bit or end with two bits $00$ ? $320$ $480$ $640$ $768$
How many bit strings of length ten either start with a $1$ bit or end with two bits $00$ ?$320$$480$$640$$768$
User Avatar
2 votes
2 votes
1 answer
3
Arjun asked Jul 2, 2019
2,790 views
UGC NET CSE | June 2019 | Part 2 | Question: 63
Consider the Euler’s phi function given by $\phi(n) = n \underset{p/n}{\Pi } \bigg( 1 – \frac{1}{p} \bigg)$ where $p$ runs over all the primes dividing $n$. What is the value of $\phi(45)$? $3$ $12$ $6$ $24$
Consider the Euler’s phi function given by$$\phi(n) = n \underset{p/n}{\Pi } \bigg( 1 – \frac{1}{p} \bigg)$$where $p$ runs over all the primes dividing $n$. What is t...
User Avatar
Link Copied!