We have sample space $S$.
$k$ events $B_{1},B_{2},…,B_{k}$ form a partition of sample space $S$. There are another event $A$ have $Pr(A)>0$.
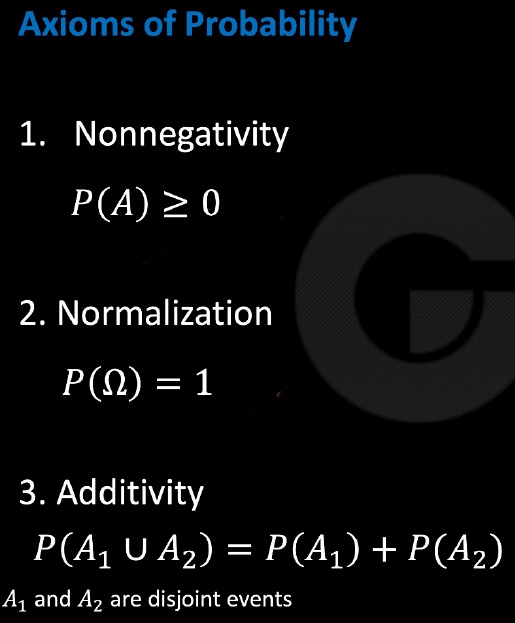
Now, according to $axioms$ $of$ $probability$ we can say that, if all of these events are $disjoint$ then we can write like this:
$P(B_{1} \cup B_{2}, … \cup B_{k}) = P(B_{1}) + P(B_{2}) + … + P(B_{k})$
So, according to our $2^{nd}$ $axiom$: $P(B_{1} \cup B_{2}, … \cup B_{k}) = 1$
Then also according to our $3^{rd}$ $axiom$ we can say that,
$P(B_{1}) + P(B_{2}) + … + P(B_{k}) = 1$
$\therefore$ $\sum_{i=1}^{k} P(B_{i}) = 1$
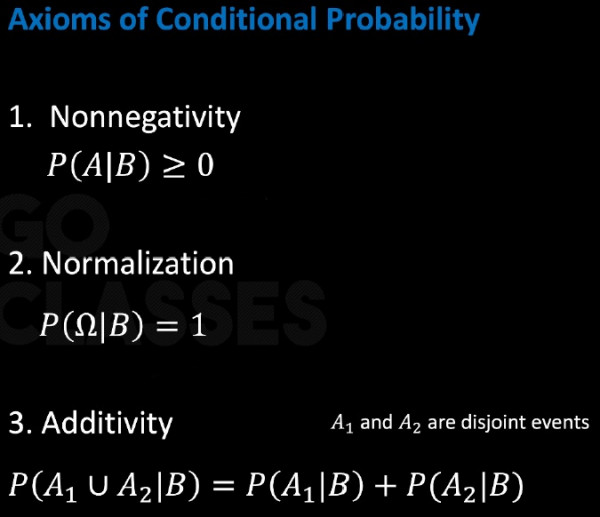
Now, Conditional probability also satisfies the $axioms$ like probability.
So, we can say that if there $A$ event also given to us then also we can get total probability summation as $1$ for the given sample space. $(Pr(A)>0)$ $[given]$
$\therefore$ $\sum_{i=1}^{k} P(B_{i} | A) = 1$ this also $valid$.
$Partition$ $Theorem$ $/$ $Total$ $Probability$
Now by these we know that if $B_{1},B_{2},…,B_{k}$ making partition in sample space for any event $A$ then,
$P(A) = \sum_{i=1}^{k} P(B_{i} \wedge A)$ $= P(A \wedge B_{1}) + P(A \wedge B_{2}) + … P(A \wedge B_{k})$
$Ans: A;B;D$
Some Resources:
$University$ $of$ $Auckland$ handbook: https://www.stat.auckland.ac.nz/~fewster/325/notes/ch2annotated.pdf [Pg. No. 23 – 26]