An even permutation is a permutation obtainable from an even number of two-element swaps, For initial set  1,2,3,4
1,2,3,4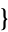 , the twelve even permutations are those with zero swaps: (
, the twelve even permutations are those with zero swaps: (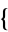 1,2,3,4
1,2,3,4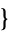 ); and those with two swaps: (
); and those with two swaps: (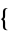 1,3,4,2
1,3,4,2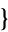 ,
, 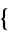 1,4,2,3
1,4,2,3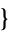 ,
, 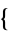 2,1,4,3
2,1,4,3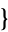 ,
, 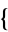 2,3,1,4
2,3,1,4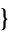 ,
, 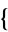 2,4,3,1
2,4,3,1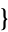 ,
, 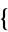 3,1,2,4
3,1,2,4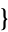 ,
, 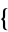 3,2,4,1
3,2,4,1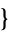 ,
, 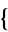 3,4,1,2
3,4,1,2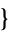 ,
, 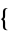 4,1,3,2
4,1,3,2 ,
,  4,2,1,3
4,2,1,3 ,
,  4,3,2,1
4,3,2,1 ). etc.
). etc.
For a set of n elements and n>2, there are n! / 2 even permutations, which is the same as the number of odd permutations