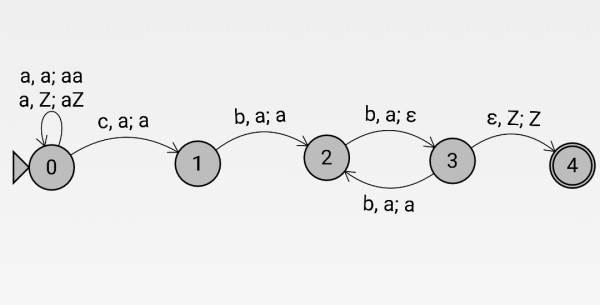
Two way to draw it
2 figure) For single input a push two a's on stack for state 0 and when c comes then don't do anything and change the state to 1 now as b will come the then top of the stack will have a then pop the a's for b's ,so when stack becomes empty then on seeing the input Null we can accept it by changing state to 2 (final) state.
1 figure) by pushing a's then don't do anything as c comes and move to state to 1 now if b comes then top of stack has a, don't do anything for first b (move to state 2)then for second b pop the a(move to state 3) repeat steps if there are more a's on the stack (as shown in figure,by moving from state 3 to state 2 ) otherwise accept it by moving from state 3 to 4.